


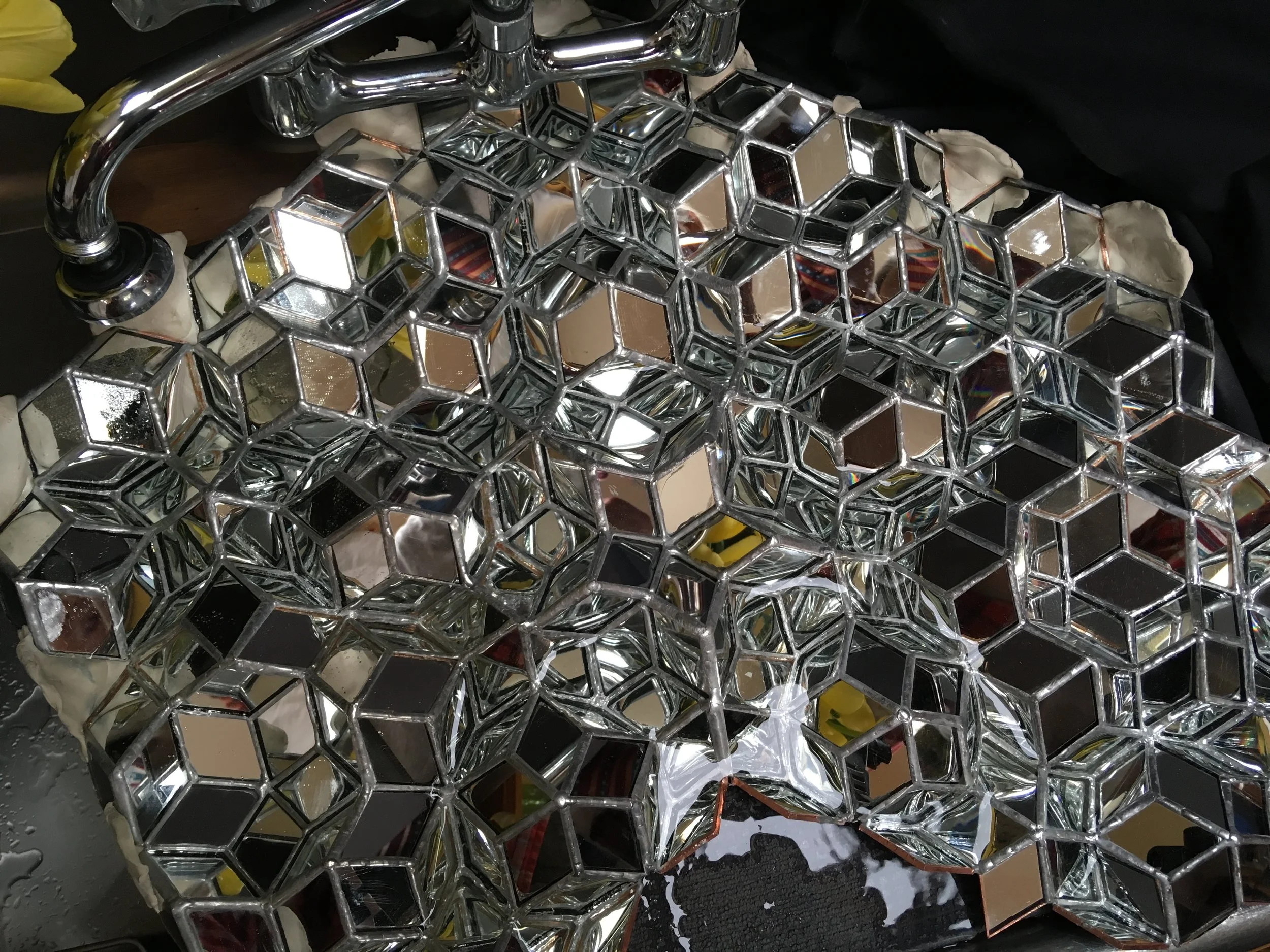



This stained glass sculpture is built from 837 identical hand-cut mirrored glass tiles edge-wrapped with copper foil and soldered together. It is reverse-coated with rubber to preserve the controlled corrosion of the mirror’s silvering. The sculpture is a Penrose tiling by rhombs raised into the third dimension. This tiling pattern is understood by mathematicians to be a projection of a plane that slices through a lattice of five-dimensional cubes. The sculpture’s geometry is characterized by the golden ratio, a proportion that occurs frequently in Nature.
Each diamond-shaped tile is a golden rhombus slanted at an angle that corresponds to a cube in five dimensions. This translates an abstract mathematical concept into
ordinary space. The structure of the sculpture correlates closely with quasi-crystalline materials that exhibit aperiodic and ‘forbidden symmetries’. Quasi-crystalline materials do not form naturally on Earth but have been found in meteorites. Their discovery sparked discussions about symmetry that continue to fuel my drive toward figuring out how quasicrystals grow in Nature.
The symmetry of regular crystalline solids are categorized into 230 allowable space groups. Scientists create X-ray diffraction patterns of space groups by reflecting a beam off the faces of a crystal’s repeating unit cells. This sculpture generates an analog version of an x-ray diffraction pattern with light reflecting out in ten different directions. As the sculpture rotates, shifting constellations of light are cast onto the ceiling in separate sheets of diamonds moving simultaneously in different directions.
In laboratories, scientists are developing quasicrystals for their potential use as invisibility shields or cloaking devices. The viewer of this sculpture cannot easily see their own reflection, or that of another standing nearby. Walking around the sculpture, rotating it, or shifting point of view will reveal unexpected sightings. These broken reflections, flickering in and out of view, demonstrate a real-time connection between observer and observed.
Sculpture by Debora Coombs
Video and editing by Jack Criddle
Music: “Storm Drain”
Todd Reynolds, Violin & Production, Ken Thomson, Bass Clarinet.
Composition by Ken Thomson (Clusterhocket Music, ASCAP/GEMA)
I am indebted to Duane Bailey for introducing me to Nicolaas De Bruijn’s 1981 paper “Algebraic theory of Penrose's non-periodic tilings of the plane.” Duane and I have built similar sculptures in cardstock to show different aspect of the underlying mathematics of aperiodic tilings.
I also wish to thank my Williams College, Massachusetts Winter Study students M. Curran, A. Flores Monge, N. Ford, E. Graf, B. Hall, J. Kim, D. Sampath, D. Smith, A. Tai, and N. Wilkinson who, in January 2018 spent hours cutting mirrored glass, wrapping diamonds with copper tape, and soldering together an early version of this sculpture.